This exercise addresses the following questions:
- How do you calculate joint forces?
- How do I calculate a frame with two fixed bearings?
- What are the different approaches to calculating joint forces?
Task
The forces F1 to F4 act on a frame with two fixed bearings and a joint. Determine the bearing loads and the forces in joint C!
F1 = F4 = 10 kN, F2 = 30 kN, F3 = 60 kN, a = 1 m
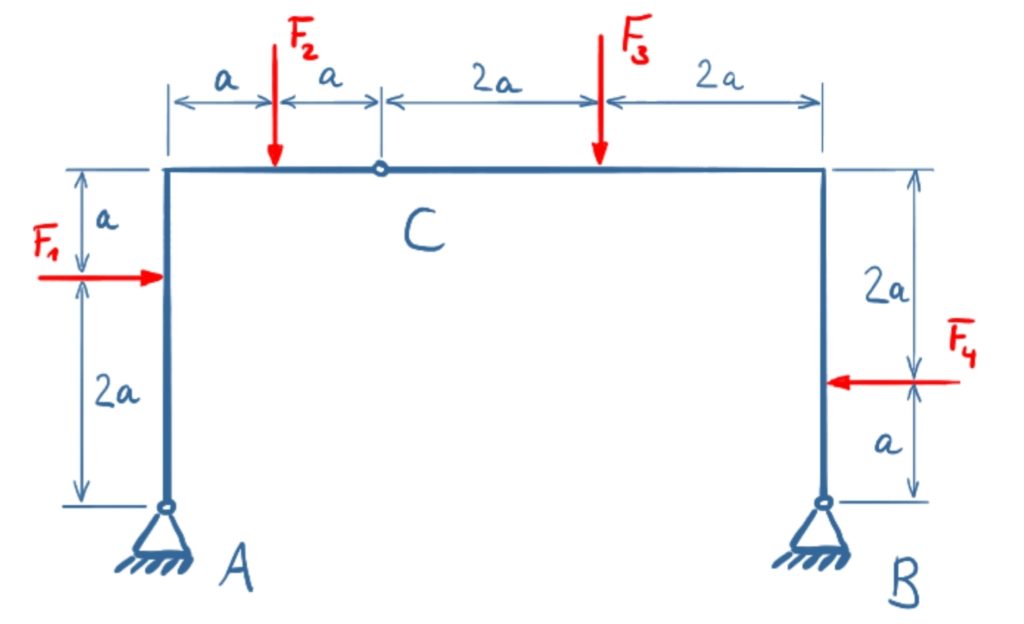
Solution
The solution can be done in different ways, here two variants are shown:
Solution variant 1 considers both areas to the left and right of the joint separately from the beginning and sets up the corresponding equations.
Solution variant 2 sets up the equilibrium conditions for the entire system as the first step, and then releases the forces of the sub-areas.
Variant 1
The following video is in German language.
The frame will be divided into two sections to determine the forces.
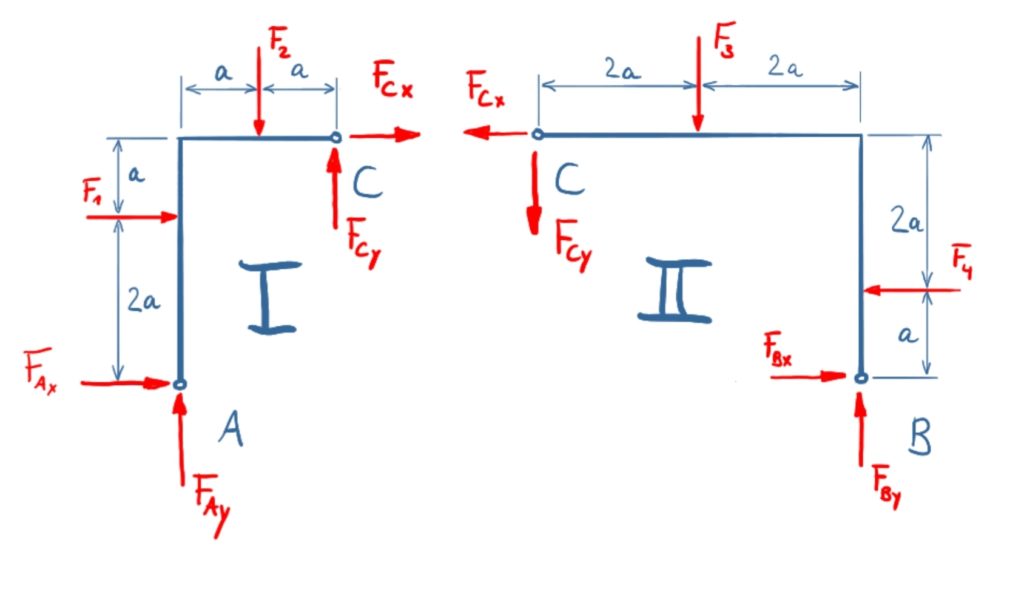
\( \DeclareMathOperator{\abs}{abs} \newcommand{\ensuremath}[1]{\mbox{$#1$}} \)
\[\tag{1} 0={F_{\mathit{Cx}}}+{F_{\mathit{Ax}}}+{F_1}\]
\[\tag{2} 0={F_{\mathit{Cy}}}+{F_{\mathit{Ay}}}-{F_2}\]
\[\tag{3} 0=2 {F_{\mathit{Cy}}} a-3 {F_{\mathit{Cx}}} a-{F_2} a-2 {F_1} a\]
\[\tag{4} 0=-{F_{\mathit{Cx}}}+{F_{\mathit{Bx}}}-{F_4}\]
\[\tag{5} 0=-{F_{\mathit{Cy}}}+{F_{\mathit{By}}}-{F_3}\]
\[\tag{6} 0=4 {F_{\mathit{Cy}}} a+3 {F_{\mathit{Cx}}} a+{F_4} a+2 {F_3} a\]
\[\tag{7} {F_{\mathit{Ax}}}=-{F_{\mathit{Cx}}}-{F_1}\]
\[\tag{8} {F_{\mathit{Cx}}}=\frac{2 {F_{\mathit{Cy}}}-{F_2}-2 {F_1}}{3}\]
\[\tag{9} {F_{\mathit{Ax}}}=-\frac{2 {F_{\mathit{Cy}}}-{F_2}-2 {F_1}}{3}-{F_1}\]
\[\tag{10} {F_{\mathit{Cy}}}=-\frac{3 {F_{\mathit{Cx}}}+{F_4}+2 {F_3}}{4}\]
\[\tag{11} {F_{\mathit{Cx}}}=\frac{-\frac{3 {F_{\mathit{Cx}}}+{F_4}+2 {F_3}}{2}-{F_2}-2 {F_1}}{3}\]
\[\tag{12} {F_{\mathit{Cx}}}=-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}\]
\[\tag{13} {F_{\mathit{Bx}}}={F_{\mathit{Cx}}}+{F_4}\]
\[\tag{14} {F_{\mathit{Bx}}}={F_4}-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}\]
\[\tag{15} {F_{\mathit{Cy}}}=-\frac{-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{3}+{F_4}+2 {F_3}}{4}\]
\[\tag{16} {F_{\mathit{By}}}={F_{\mathit{Cy}}}+{F_3}\]
\[\tag{17} {F_{\mathit{By}}}=\frac{-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{3}+{F_2}+2 {F_1}}{2}+{F_3}\]
\[\tag{18} {F_{\mathit{Ax}}}=\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}-{F_1}\]
\[\tag{19} {F_{\mathit{Ay}}}={F_2}-{F_{\mathit{Cy}}}\]
\[\tag{20} {F_{\mathit{Ay}}}={F_2}-\frac{-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{3}+{F_2}+2 {F_1}}{2}\]
\[\tag{21} {F_{\mathit{Ax}}}=\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}-{F_1}\]
\[\tag{22} {F_{\mathit{Ay}}}={F_2}-\frac{-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{3}+{F_2}+2 {F_1}}{2}\]
\[\tag{23} {F_{\mathit{Bx}}}={F_4}-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}\]
\[\tag{24} {F_{\mathit{By}}}=\frac{-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{3}+{F_2}+2 {F_1}}{2}+{F_3}\]
\[\tag{25} {F_{\mathit{Cx}}}=-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}\]
\[\tag{26} {F_{\mathit{Cy}}}=\frac{-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{3}+{F_2}+2 {F_1}}{2}\]
\[\tag{27} {F_{\mathit{Ax}}}=15.56 \mathit{kN}\]
\[\tag{28} {F_{\mathit{Ay}}}=43.33 \mathit{kN}\]
\[\tag{29} {F_{\mathit{Bx}}}=-15.56 \mathit{kN}\]
\[\tag{30} {F_{\mathit{By}}}=46.67 \mathit{kN}\]
\[\tag{31} {F_{\mathit{Cx}}}=-25.56 \mathit{kN}\]
\[\tag{32} {F_{\mathit{Cy}}}=-13.33 \mathit{kN}\]
Variant 2
This solution is calculated here exclusively with the variables, since the numerical values have already been calculated above.
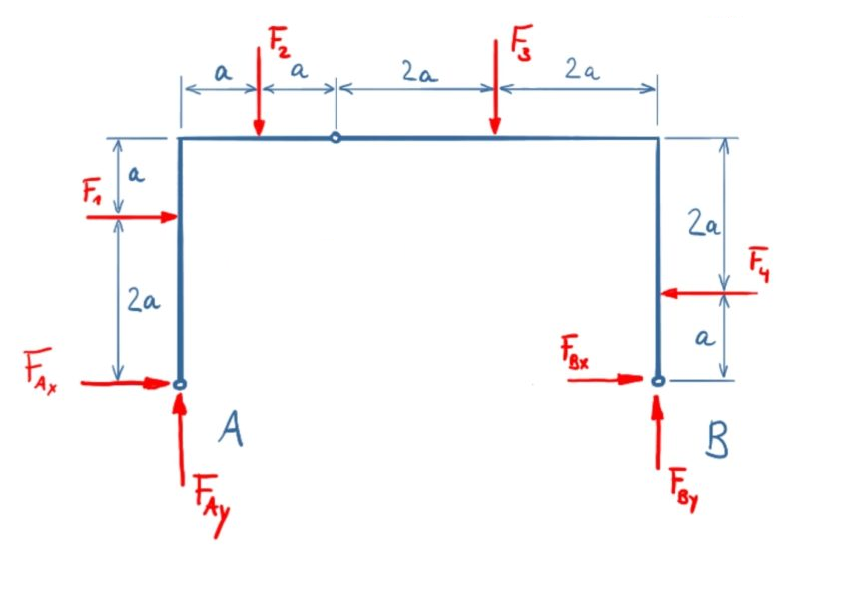
\( \DeclareMathOperator{\abs}{abs} \newcommand{\ensuremath}[1]{\mbox{$#1$}} \)
Establishing the balance of forces and moments for the entire system
\[\tag{1} \sum F_x = 0={F_{\mathit{Bx}}}+{F_{\mathit{Ax}}}-{F_4}+{F_1}\]
\[\tag{2} \sum F_y = 0={F_{\mathit{By}}}+{F_{\mathit{Ay}}}-{F_3}-{F_2}\]
\[\tag{3} \sum M(A) = 0=6 {F_{\mathit{By}}} a+{F_4} a-4 {F_3} a-{F_2} a-2 {F_1} a\]
The vertical support reaction FBy can thus be calculated directly:
\[\tag{4} {F_{\mathit{By}}}=-\frac{{F_4}-4 {F_3}-{F_2}-2 {F_1}}{6}\]
FBy is now substituted into equation 1, whereby FAy follows.
\[\tag{5} 0={F_{\mathit{Ay}}}-\frac{{F_4}-4 {F_3}-{F_2}-2 {F_1}}{6}-{F_3}-{F_2}\]
\[\tag{6} {F_{\mathit{Ay}}}=\frac{{F_4}+2 {F_3}+5 {F_2}-2 {F_1}}{6}\]

Next, the balance of forces in the y-direction is set up for section I in order to calculate the joint force FCy:
\( \DeclareMathOperator{\abs}{abs} \newcommand{\ensuremath}[1]{\mbox{$#1$}} \)
\[\tag{7} \sum F_y = 0={F_{\mathit{Cy}}}+{F_{\mathit{Ay}}}-{F_2}\]
\[\tag{8} {F_{\mathit{Cy}}}=-\frac{{F_4}+2 {F_3}-{F_2}-2 {F_1}}{6}\]
The sum of moments around A leads to FCx.
\[\tag{9} \sum M(A) = 0=2 {F_{\mathit{Cy}}} a-3 {F_{\mathit{Cx}}} a-{F_2} a-2 {F_1} a\]
\[\tag{10} {F_{\mathit{Cx}}}=-\frac{{F_4}+2 {F_3}+2 {F_2}+4 {F_1}}{9}\]
The equilibrium of forces in the x-direction for section I results in the horizontal support reaction in A.:
\[\tag{11} 0={F_{\mathit{Cx}}}+{F_{\mathit{Ax}}}+{F_1}\]
\[\tag{12} {F_{\mathit{Ax}}}=\frac{{F_4}+2 {F_3}+2 {F_2}-5 {F_1}}{9}\]
FAx is now substituted into equation 2, whereby FBx follows.
\[\tag{13} {F_{\mathit{Bx}}}=\frac{8 {F_4}-2 {F_3}-2 {F_2}-4 {F_1}}{9}\]
The second solution leads to the desired results faster!

