In this exercise, the bending line for a beam with partial line load, individual force and moment is calculated.
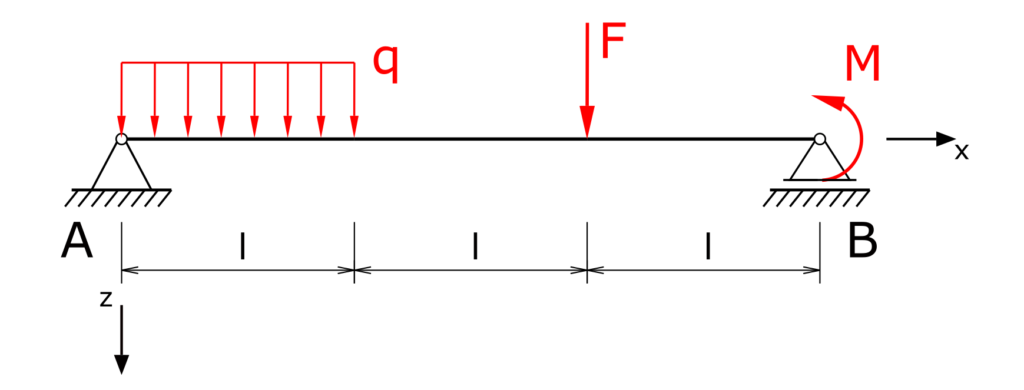
Task
A beam on a fixed bearing and a floating bearing is loaded over the length l by the line load q. Additionally the force F acts at 2l and the moment M on the floating bearing B. The bending line of the beam has to be determined.
Solution
In the first step, the equations for the bearing reactions are determined. To determine the internal forces, the beam is divided into three sections. The horizontal forces are not considered further in the following equations, since they are obviously zero. Left turning moments are positive.
Bearing loads
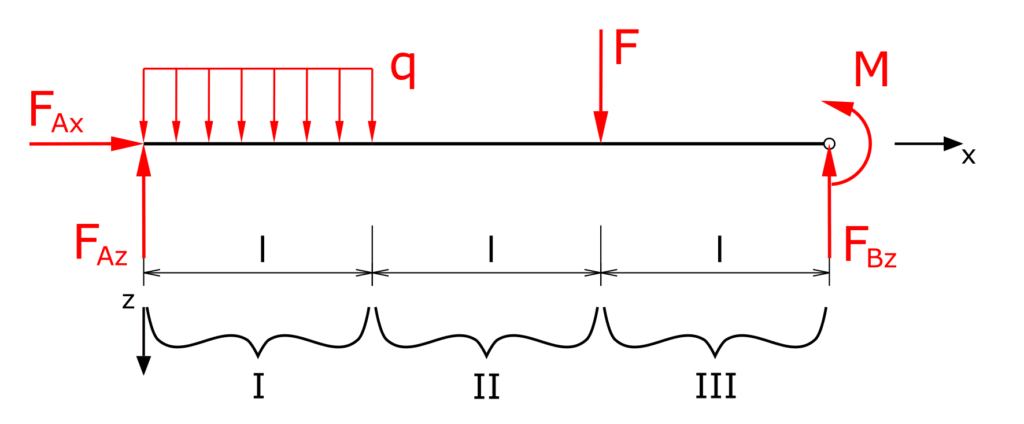
The sum of forces in z-direction gives
\[ \tag{1} \sum F_z = 0 = -F_{Az} - F_{Bz} + F + \int_0^{l}{qdx} \]
\[ \tag{2} 0 = -F_{Az} - F_{Bz} + F + q \cdot l \]
The sum of moments regarding point A
\[ \tag{3} \sum M(A) = 0 = 3 \cdot F_{Bz} \cdot l - 2 \cdot F \cdot l - \int_0^{l}{qxdx} + M \]
\[ \tag{4} 0 = 3 \cdot F_{Bz} \cdot l - 2 \cdot F \cdot l - \frac{1}{2} q \cdot l^2 + M \]
Both bearing loads FAz and FBz can now be solved. They are:
\[ \tag{5} {F_{\mathit{Bz}}}=\frac{{{l}^{2}} q+4 F l-2 M}{6 l} \]
\[ \tag{6} {F_{\mathit{Az}}}=\frac{5 {{l}^{2}} q+2 F l+2 M}{6 l}\]
The next step is to determine the internal forces in sections I to III. In order to be able to integrate the function of the line load from 0 to x, it is formulated as a function of the auxiliary coordinate ξ. Normal forces are denoted by N, transverse forces by Q and bending moments by Mb.
Determination of the internal forces
Section I
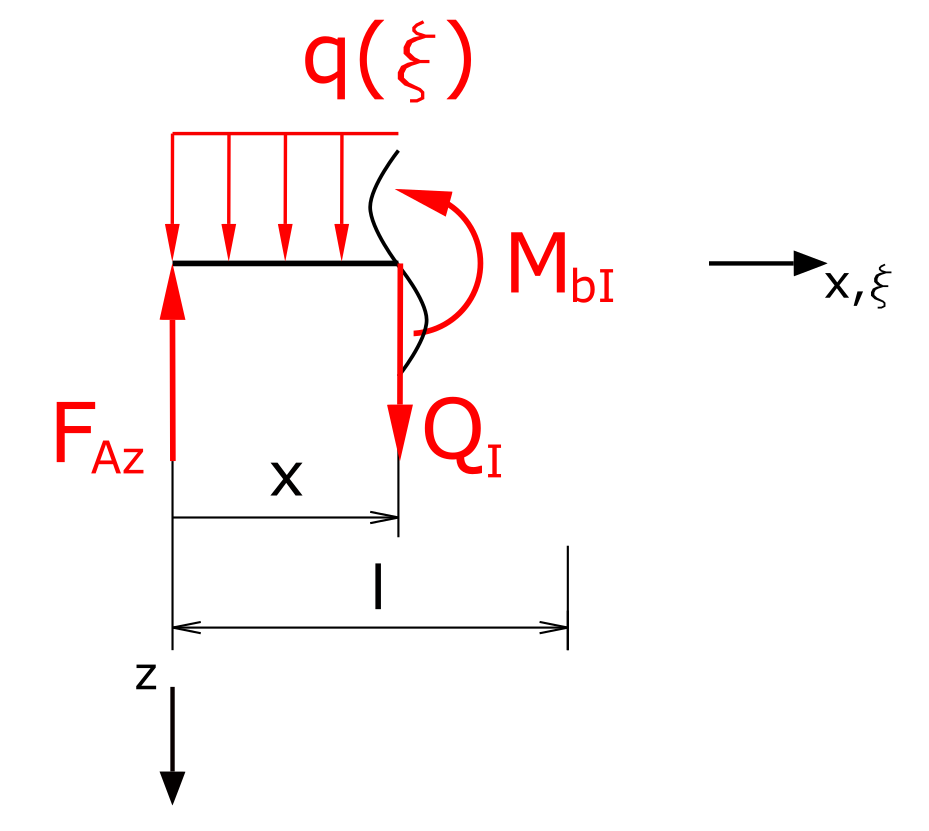
The sum of forces in z-direction gives
\[ \tag{7} \sum F_z = 0 = Q_I - F_{Az} + \int_0^{x}{qd\xi} \]
\[ \tag{8} {Q_I}=-\frac{6 l q x-5 {{l}^{2}} q-2 F l-2 M}{6 l} \]
The sum of moments regarding point x
\[ \tag{9} \sum M(x) = 0 = \int_0^{x}{q (x-\xi) d \xi} - F_{Az} \cdot x + M_{bI} \]
\[ \tag{10} {M_{\mathit{bI}}}=-\frac{3 l q\, {{x}^{2}}+\left( -5 {{l}^{2}} q-2 F l-2 M\right) x}{6 l}\]
Section II
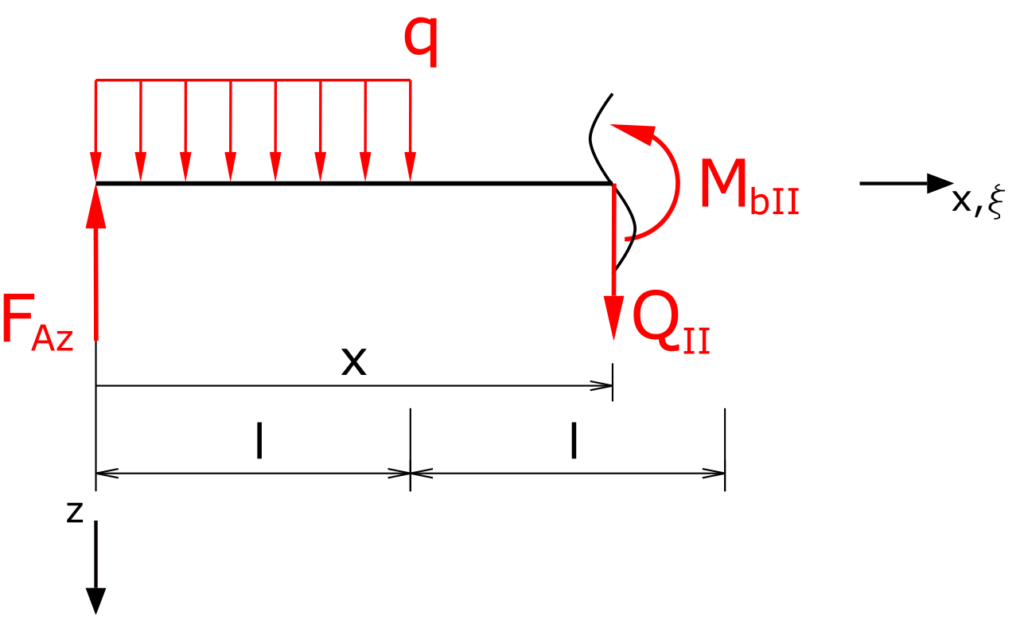
The sum of forces in z-direction gives:
\[ \tag{11} \sum F_z = 0 = Q_{II} - F_{Az} + \int_0^{l}{q d \xi} \]
\[ \tag{12} {Q_{\mathit{II}}}=-\frac{{{l}^{2}} q-2 F l-2 M}{6 l}\]
The sum of moments regarding point x
\[ \tag{13} \sum M(x) = 0 = \int_0^{l}{q (x - \xi) d \xi} - F_{Az} \cdot x + M_{bII} \]
\[ \tag{14} {M_{\mathit{bII}}}=-\frac{\left( {{l}^{2}} q-2 F l-2 M\right) x-3 {{l}^{3}} q}{6 l}\]
Section III
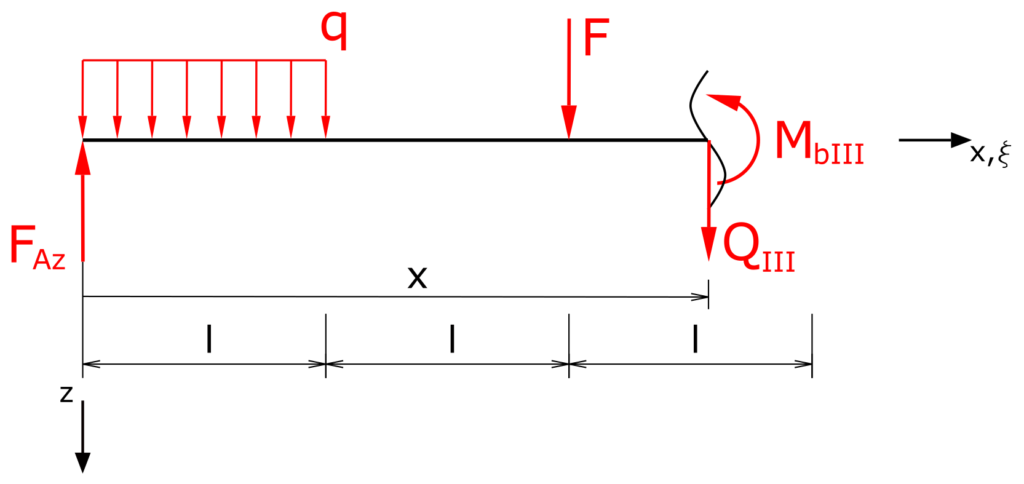
The sum of forces in z-direction gives
\[ \tag{15} \sum F_z = 0 = Q_{III} - F_{Az} + F + \int_0^{l}{q d \xi} \]
\[ \tag{16} {Q_{\mathit{III}}}=-\frac{{{l}^{2}} q+4 F l-2 M}{6 l}\]
The sum of moments regarding point x
\[ \tag{17} \sum M(x) = 0 = \int_0^{l}{q (x - \xi) d \xi} - F_{Az} \cdot x + F \cdot (x - 2 \cdot l) + M_{bIII} \]
\[ \tag{18} {M_{\mathit{bIII}}}=-\frac{\left( {{l}^{2}} q+4 F l-2 M\right) x-3 {{l}^{3}} q-12 F\, {{l}^{2}}}{6 l}\]
The next step is the determination of the bending line functions.
Bending line
The function of the bending line is designated as w, or the first derivative as w' and the second derivative as w''. The modulus of elasticity is E and the area moment of inertia is I.
Section I
\[ \tag{19} {M_{\mathit{bI}}}=-\frac{3 l q\, {{x}^{2}}+\left( -5 {{l}^{2}} q-2 F l-2 M\right) x}{6 l} \]
\[ \tag{20} w''_I = - \frac{1}{E \cdot I} M_{bI} \]
\[ \tag{21} w''_I = -\frac{-3 l q\, {{x}^{2}}-\left( -5 {{l}^{2}} q-2 F l-2 M\right) x}{6 E I l}\]
\[ \tag{22} w'_I = {c_1}-\frac{\frac{\left( 5 {{l}^{2}} q+2 F l+2 M\right) \, {{x}^{2}}}{2}-l q\, {{x}^{3}}}{6 E I l}\]
\[ \tag{23} w_I = -\frac{\frac{\left( 5 {{l}^{2}} q+2 F l+2 M\right) \, {{x}^{3}}}{6}-\frac{l q\, {{x}^{4}}}{4}}{6 E I l}+{c_1} x+{c_2}\]
Section II
\[ \tag{24} {M_{\mathit{bII}}}=-\frac{\left( {{l}^{2}} q-2 F l-2 M\right) x-3 {{l}^{3}} q}{6 l}\]
\[ \tag{25} w''_{II} = - \frac{1}{E \cdot I} M_{bII} \]
\[ \tag{26} w''_{II} = -\frac{3 {{l}^{3}} q-\left( {{l}^{2}} q-2 F l-2 M\right) x}{6 E I l}\]
\[ \tag{27} w'_{II} = {c_3}-\frac{\frac{\left( -{{l}^{2}} q+2 F l+2 M\right) \, {{x}^{2}}}{2}+3 {{l}^{3}} q x}{6 E I l} \]
\[ \tag{28} w_{II} = -\frac{\frac{\left( -{{l}^{2}} q+2 F l+2 M\right) \, {{x}^{3}}}{6}+\frac{3 {{l}^{3}} q\, {{x}^{2}}}{2}}{6 E I l}+{c_3} x+{c_4}\]
Section III
\[ \tag{29} {M_{\mathit{bIII}}}= -\frac{\left( {{l}^{2}} q+4 F l-2 M\right) x-3 {{l}^{3}} q-12 F\, {{l}^{2}}}{6 l}\]
\[ \tag{30} w''_{III} = - \frac{1}{E \cdot I} M_{bIII} \]
\[ \tag{31} w''_{III} = -\frac{-\left( {{l}^{2}} q+4 F l-2 M\right) x+3 {{l}^{3}} q+12 F\, {{l}^{2}}}{6 E I l}\]
\[ \tag{32} w'_{III} = {c_5}-\frac{\frac{\left( -{{l}^{2}} q-4 F l+2 M\right) \, {{x}^{2}}}{2}+3 {{l}^{3}} q x+12 F\, {{l}^{2}} x}{6 E I l}\]
\[ \tag{33} w_{III} = -\frac{\frac{\left( -{{l}^{2}} q-4 F l+2 M\right) \, {{x}^{3}}}{6}+\frac{3 {{l}^{3}} q\, {{x}^{2}}}{2}+6 F\, {{l}^{2}}\, {{x}^{2}}}{6 E I l}+{c_5} x+{c_6}\]
The constants of integration are determined via the boundary and secondary conditions.
Boundary and secondary conditions
The deflection at x = 0 is zero.
\[ \tag{34} w_I(x=0)=0=\mathit{c_2} \]
The deflection at the point x = l is the same for bending line I and bending line II.
\[ \tag{35} w_I(x=l)=w_{II}(x=l) \]
\[ \tag{36} -\frac{\frac{{{l}^{3}}\, \left( 5 {{l}^{2}} q+2 F l+2 M\right) }{6}-\frac{{{l}^{5}} q}{4}}{6 E I l}+{c_1} l+{c_2}=-\frac{\frac{{{l}^{3}}\, \left( -{{l}^{2}} q+2 F l+2 M\right) }{6}+\frac{3 {{l}^{5}} q}{2}}{6 E I l}+{c_3} l+{c_4} \]
The inclination at the point x = l is the same for bending line I and bending line II.
\[ \tag{37} w'_I(x=l)=w'_{II}(x=l) \]
\[ \tag{38} {c_1}-\frac{\frac{{{l}^{2}}\, \left( 5 {{l}^{2}} q+2 F l+2 M\right) }{2}-{{l}^{4}} q}{6 E I l}={c_3}-\frac{\frac{{{l}^{2}}\, \left( -{{l}^{2}} q+2 F l+2 M\right) }{2}+3 {{l}^{4}} q}{6 E I l} \]
The deflection at the point x = 2l is the same for bending line II and bending line III.
\[ \tag{39} w_{II}(x=2l)=w_{III}(x=2l) \]
\[ -\frac{\frac{4 {{l}^{3}}\, \left( -{{l}^{2}} q+2 F l+2 M\right) }{3}+6 {{l}^{5}} q}{6 E I l}+2 {c_3} l+{c_4}=... \]
\[ \tag{40} ...=-\frac{\frac{4 {{l}^{3}}\, \left( -{{l}^{2}} q-4 F l+2 M\right) }{3}+6 {{l}^{5}} q+24 F\, {{l}^{4}}}{6 E I l}+2 {c_5} l+{c_6} \]
The inclination at the point x = 2l is the same for bending line II and bending line III.
\[ \tag{41} w'_{II}(x=2l)=w'_{III}(x=2l) \]
\[ {c_3}-\frac{2 {{l}^{2}}\, \left( -{{l}^{2}} q+2 F l+2 M\right) +6 {{l}^{4}} q}{6 E I l}=... \]
\[ \tag{42} ...={c_5}-\frac{2 {{l}^{2}}\, \left( -{{l}^{2}} q-4 F l+2 M\right) +6 {{l}^{4}} q+24 F\, {{l}^{3}}}{6 E I l} \]
The deflection at the point x = 3l is zero.
\[ \tag{43} w_{III}(x=3l)=0 \]
\[ \tag{44} 0 = -\frac{\frac{9 {{l}^{3}}\, \left( -{{l}^{2}} q-4 F l+2 M\right) }{2}+\frac{27 {{l}^{5}} q}{2}+54 F\, {{l}^{4}}}{6 E I l}+3 {c_5} l+{c_6} \]
The unknowns can now be resolved.
\[ \tag{45} {c_1}=\frac{25 {{l}^{3}} q+32 F\, {{l}^{2}}+36 M l}{72 E I}\]
\[ \tag{46} {c_2}=0\]
\[ \tag{47} {c_3}=\frac{37 {{l}^{3}} q+32 F\, {{l}^{2}}+36 M l}{72 E I}\]
\[ \tag{48} {c_4}=-\frac{{{l}^{4}} q}{24 E I}\]
\[ \tag{49} {c_5}=\frac{37 {{l}^{3}} q+176 F\, {{l}^{2}}+36 M l}{72 E I}\]
\[ \tag{50} {c_6}=-\frac{{{l}^{4}} q+32 F\, {{l}^{3}}}{24 E I}\]
Finally, the three functions of the bending lines can now be described as follows:
\[ \tag{51} w_I(x) = \frac{\left( 25 {{l}^{3}} q+32 F\, {{l}^{2}}+36 M l\right) x}{72 E I}-\frac{\frac{\left( 5 {{l}^{2}} q+2 F l+2 M\right) \, {{x}^{3}}}{6}-\frac{l q\, {{x}^{4}}}{4}}{6 E I l}\]
\[ \tag{52} w_{II}(x) = -\frac{\frac{\left( -{{l}^{2}} q+2 F l+2 M\right) \, {{x}^{3}}}{6}+\frac{3 {{l}^{3}} q\, {{x}^{2}}}{2}}{6 E I l}+\frac{\left( 37 {{l}^{3}} q+32 F\, {{l}^{2}}+36 M l\right) x}{72 E I}-\frac{{{l}^{4}} q}{24 E I}\]
\[ w_{III}(x) = -\frac{\frac{\left( -{{l}^{2}} q-4 F l+2 M\right) \, {{x}^{3}}}{6}+\frac{3 {{l}^{3}} q\, {{x}^{2}}}{2}+6 F\, {{l}^{2}}\, {{x}^{2}}}{6 E I l}+\frac{\left( 37 {{l}^{3}} q+176 F\, {{l}^{2}}+36 M l\right) x}{72 E I}... \]
\[ \tag{53} ...-\frac{{{l}^{4}} q+32 F\, {{l}^{3}}}{24 E I} \]
The determination of the bending lines is now complete. It can be clearly seen that using the same coordinate origin in sections I to III results in very extensive formulas. A simplification can be achieved, for example, by introducing a substitute coordinate in the third area, which runs in the opposite direction to the original x-direction and starts at x = 3l.

